A few months ago, I stumbled on an article about a physicist who believes octonion math can underly physics. It was a pretty interesting article in its own right, but what really grabbed me at the time was the diagram near the end, giving a brief explainer of complex, quaternion, and octonion math:

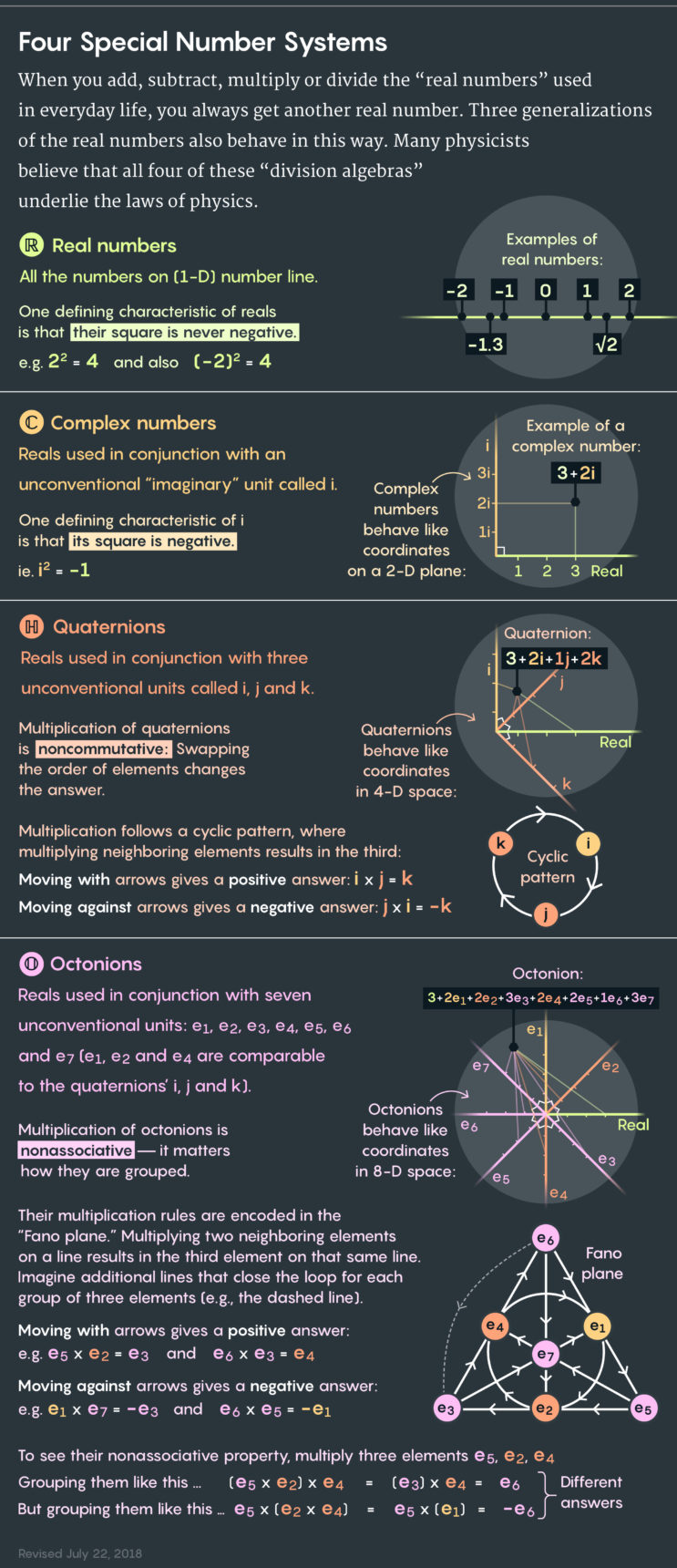
Tho I'd seen quaternion and octonion math before, I'd never quite seen the quaternion units depicted in that rock-paper-scissors diagram. And then when I looked down at the octonion diagram of the unit interactions, I was struck: that looks like a (simple) goetic diagram! The sort of "mystic circles and triangles with some latin bullshit" thing you see in anime alchemy and other magic.

I couldn't get this out of my head, and after some noodling on Twitter (link goes to the bottom of the thread to ensure you see the whole thing, scroll to the top to start reading) and chatting with my brother, I'd come up with a whole little overcomplicated elemental transmutation alchemy system which I really liked.

Here's the diagram in Google Diagrams. (Sorry, I haven't spent the time to make it an SVG yet.)

lrn 2 alchemy nub

Reading the diagram requires a little explanation.

First, the circle, and each of the six straight lines (with the end looping back to the beginning to make a circle as well) describe seven transmutation cycles; the elements in each cycle can be transmuted into each other.

To do so, you take an element from one "node", the source, and an element from another node, the catalyst. This will produce one of the two elements in the third node of the cycle. Which one depends on the chirality of your alchemy: if your source and catalyst are both the "top" elements of their nodes, and tracing the diagram from source to catalyst follows the arrow connecting them, then the result is the top element of the final node. For example, fire catalyzed with water produces lightning; fire catalyzed with life loops around and produces aether; etc.

However, choosing the "bottom" element in a node inverts the result: fire catalyzed with air (inverted water) produces earth. Running the transmutation in reverse also inverts the result: water catalyzed with fire (inverted order) produces earth. Two inversions goes back to normal: ice catalyzed with air (both inverted, normal order) produces lightning; so does water catalyzed with ice (one inverted, inverted order). All three inversions (both elements from the "bottom", with the reaction run against the arrows) is back to inverting: air catalyzed with ice produces earth.

There's a hidden node in here, too, representing non-elemental substance. (In octonions, it's the value 1; the real unit, as opposed to the 7 complex units e₁-e₇.) By default it's mundane; a lack of magic. Inverted, it represents wild magic, destructive and reactive without the guiding template of an element constraining it. You can produce these by choosing the source and catalyst from the same node: if they're opposing elements, like fire and ice, they produce mundanity as the elements cancel out (regardless of source/catalyst order); if they're the same element, like fire and fire, they produce wild magic as the element burn themselves out from the clash but leave behind their energy.

Mundanity combined with anything produces the other thing: mundane catalyzed with fire produces fire, wild magic catalyzed with mundane produces wild magic, etc. Wild magic combined with anything inverts the other: fire + wild magic produces ice, wild magic + wild magic produces mundanity, etc.

(This, if it's not obvious, perfectly replicates octonion math, if you're only allowed to use the eight units, their negatives, and multiplication.)

And the point?

I imagine that in a video game using these, a character aligned with an element would do more damage with and take less damage from attacks of that element, but take more damage from attacks with the opposing element. Aligning with mundane gives you weaker attacks, but a generalized minor defense against all elements; aligning with wild magic gives you stronger attacks, but a generalized minor weakness against all elements. There'd probably be some environmental interactions, too, like using fire abilities on a fire-aligned hex would give some bonus, etc.

I could also imagine a game starting with just the central circle, representing the "classical" elements, and perhaps the druidic wood/metal center. You don't know about the triangle points, so you can't use the druidic elements in alchemy, except for using wild magic to invert it. Then, later in the game, you meet some people from a different magical tradition that introduce you to the "astral" elements (the three on the triangle points), and the rest of alchemy unfolds - you can finally use the druidic element in alchemy!

Other than that, eh, this is just a fun thing to think about. Thank you, complicated mathematical structure, for giving me just enough rules to wrap some interesting stories around.